
A spacecraft is in a clockwise circular orbit about the sun, near the counterclockwise circular orbit of the earth.
Getting a boost from a collision
Go to an optional historical introduction about the Galileo spacecraft.
How do you bounce a spacecraft off a planet?
Let's take a closer look at how a gravity assist works. If you only look at half of the picture, it all seems pretty straightforward. If you drop a spacecraft into a planet, the gravitational attraction of the planet speeds it up, just like a dropped ball speeds up on its way to the floor. Let's assume that you've very carefully aimed your spacecraft so that it doesn't actually hit the planet. Instead, it executes a near-miss. (An interesting aside here: that's basically what an orbit is. A near miss that goes on and on and on. A spacecraft in orbit is always falling toward the planet, but always missing.)
The spacecraft misses the planet, but doesn't get to keep that speed it gained. After the spacecraft races past the planet, gravity slows it down on the way out, just as the ball slowed down on its way up. In the end the spacecraft leaves the planet with the same speed with which it arrived.
How can we set this up so that passing the planet gives the spacecraft a boost? To figure out what's going on, you can visit the experiment in which two balls are dropped together and bounce off the floor with one ball gaining energy from the other and rocketing into the air. Bouncing Ball Experiment. This experiment is a three body collision problem, the two balls plus the earth. The spacecraft-earth-sun is also a three body problem.
Let's say you've got a spacecraft that's orbiting the sun at the same distance as the earth. The spacecraft is traveling in the opposite direction as the earth - the earth orbits counterclockwise, and the spacecraft orbits clockwise. Both are going at a speed of V1 = 30 kilometers/second. The spacecraft comes in towards the planet, swings around it in a cosmic do-se-do, and leaves moving out along the line of its approach. That's your planetary collision. A collision without touching.

The spacecraft and planet come together at 2V1 = 60 kilometers/second and leave each other at 60 kilometers/second. That's what you see if you are standing on the earth.
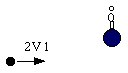
But suppose you back up and look at the collision in the frame of the distant stars. Then you see a spacecraft initially orbiting the sun at 30 kilometers/second. After the collision, you see a spacecraft going 90 kilometers/second! The spacecraft is leaving the earth at 60 kilometers/second and the earth is going 30 kilometers/second so 60 + 30 = 90! That's fast enough to give the spacecraft escape velocity from the sun , heading out toward interstellar space along a hyperbolic trajectory
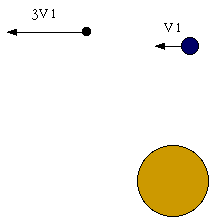
The spacecraft gains kinetic energy in this encounter. Where does that energy come from? The encounter with Galileo slowed the earth down. Not by much, of course. When the Galileo spacecraft swung by earth, it sped up by over 16,000 kilometers per hour with respect to the sun, and the earth slowed down by 10 billionths of a centimeter per year.
The Galileo spacecraft.Back in the early 1980's NASA had a problem. They had been planning to launch the Galileo spacecraft to Jupiter. In NASA's original plan, the spacecraft was to be propelled directly toward Jupiter by a powerful Centaur rocket. That rocket was to have been carried into orbit by the space shuttle. It was a fine plan--until the Challenger disaster in January of 1986. After that explosion, NASA reexamined the safety of carrying a liquid-fueled rocket inside the shuttle and decided that the risk was not acceptable. They began to investigate other alternatives.
They could launch the Galileo using the weaker, safer solid-fueled IUS (Inertial Upper Stage). But the IUS could not provide enough thrust to overcome the pull of the sun's gravity and propel the spacecraft to Jupiter. What a dilemma! To solve it, NASA drew on the ideas of a mathematics graduate student at the University of California at Los Angeles: Michael Minovitch. We don't know if Minovitch ever dropped basketballs and tennis balls together, but his ideas relied on some of the same principles. To get a feel for Minovitch's idea, think of the Galileo spacecraft as a table tennis ball and the planet of Venus as a basketball. What NASA did, in essence, is bounce the Galileo spacecraft off of Venus to give it a velocity boost. Then NASA bounced the spacecraft off of the earth twice to send it shooting toward Jupiter, a maneuver known as a "gravity assist."
|
Scientific Explorations with Paul Doherty |
|
21 August 2003 |